Subdivisions

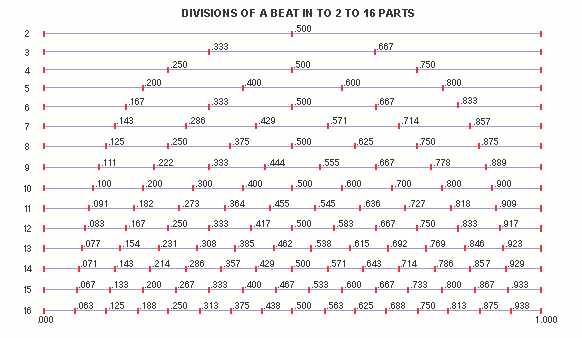
This chart graphs the divisions of a beat from 2 to 16 parts.

A beat may be of any value i.e. etc. at any metronomic rate.

The beat begins at the left at 0.000 and ends at the right at 1.000.

Each line is numbered at the left, beginning with the number 2. These numbers represent the number of attacks on that specific line.

The attack of the first note always occurs at 0.000. The tick marks along the horizontal lines plot the subsequent divisions. The 3-digit decimals to the right of each tick mark provide the arithmetic place of occurrence for that tick.

As an example, for the first line, division by two, the first attack occurs at 0.000. If the beat is a , the second occurs at 0.500.

For the next line, division by three, the first attack occurs at 0.000. If the beat is a , the next occurs at 0.333; the third at 0.667.

The same process applies to all the remaining lines of the chart.

The chart may be used to provide answers to the following questions:

a) given two attacks X and Y, which one occurs first?

b) what is the duration between attacks?

As an example, assume that there are two players, one of whom (X) must play ;

the other (Y) must play ; which one comes first?

While it is certainly possible to calculate the fractions, it may prove far easier for question a) above, and certainly for question b), to proceed as follows:

in order to answer a) - (which one occurs first) X locates line #5, and counts off the first three ticks which represent the , and determines that the next tick (which represents the start of the final occurs at .600.

Similarly, Y locates line #7, and after counting off the first four ticks, determines that the last occurs at .571.

In order to answer b) - (what is the duration between attacks) - one subtracts the two values (.600 -.571) to discover that Y (the septuplet person) plays .029 of a beat earlier than X (the quintuplet person).

Unfortunately, that is not the end of it. It is all very well to know that Y proceeds X by .029 of a beat, but does that have any practical meaning? Can that difference be perceived (?), for if not, there is no point wasting the time trying to achieve it.

The threshold at which the attacks of two events cannot be individually distinguished, but rather are perceived as if they were simultaneous is (crudely speaking, but sufficient for our practical purposes) approximately 1/20 of a second, or 50 msec. That is to say, if two attacks are less than 50 msec. apart they are heard as a single attack, and that under laboratory conditions - i.e. if all hell is breaking loose, the threshold may be much higher. For our example above, in order to determine significance, question c) must be answered:

c) is a difference value of .029 of a beat greater or less than 50 msec. ?(for if less than 50 msec. we can dispense with the distinction).

The answer to c) depends entirely upon the speed, or metronome mark, of the beat.

If the beat =	and the threshold of simultaneity always =	A difference value less than .XXX is not perceptible
30 MM (2000 msec)	50 msec	.025
60 MM (1000 " )	50 msec	.050
120 MM (500 " )	50 msec	.100
240 MM (250 " )	50 msec	.200

It can be seen from this table that, if the tempo is somewhere close to 30 MM, a difference of .029 of a beat is just at the threshold of audibility. Any tempo substantially faster than 30 MM has a threshold value which is greater than .029, and therefore, the difference of .029 posed in the above example is too small to matter, i.e. it is not audibly significant if one's only concern is arithmetic exactitude.

Why have I not provided far more detail for the difference limit values at a myriad of tempi?

The answer is that man does not live (or interpret music) by difference limits alone. By this I mean the following: imagine a situation where the composer has at least two voices at different rates of speed, one rate being notated as quintuplets, the other as septuplets. There may very well occur situations where, even though the two attacks are nominally supposed to be absolutely together, or are well within the difference threshold, one thinks it imperative - for structural, motivic, phrasal, rhythmic shape or other reasons, to exaggerate the difference between the two attacks, even to the point of distorting the actual arithmetic values. There is no one-size-fits-all answer to these situations. One weighs up the evidence; considers what the composer is trying, and has previously tried, to convey; adds one's personal recollections of other similar or dissimilar problems; and decides a judgment that may or may not be later reversed, but for now, will have to do.

In other words, once again one tries to steer - carefully - between the Scylla and Charybdis of Stravinsky's "The sin against the spirit of the work always begins with a sin against its letter" and Busoni's "What the composer's inspiration necessarily loses through notation, his interpreter should restore by his own."

Paul Zukofsky

March 2003